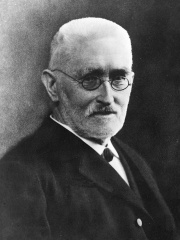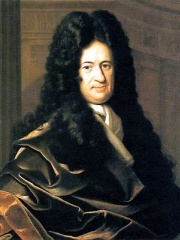
The Most Famous

MATHEMATICIANS from Germany

This page contains a list of the greatest German Mathematicians. The pantheon dataset contains 1,004 Mathematicians, 93 of which were born in Germany. This makes Germany the birth place of the 3rd most number of Mathematicians behind United Kingdom, and France.

Top 10

The following people are considered by Pantheon to be the top 10 most legendary German Mathematicians of all time. This list of famous German Mathematicians is sorted by HPI (Historical Popularity Index), a metric that aggregates information on a biography’s online popularity. Visit the rankings page to view the entire list of German Mathematicians.

1. Carl Friedrich Gauss (1777 - 1855)

With an HPI of 87.70, Carl Friedrich Gauss is the most famous German Mathematician. His biography has been translated into 154 different languages on wikipedia.

Johann Carl Friedrich Gauss (German: Gauß [kaʁl ˈfʁiːdʁɪç ˈɡaʊs] ; Latin: Carolus Fridericus Gauss; 30 April 1777 – 23 February 1855) was a German mathematician, astronomer, geodesist, and physicist who contributed to many fields in mathematics and science. He was director of the Göttingen Observatory and professor of astronomy from 1807 until his death in 1855. He is widely considered one of the greatest mathematicians ever. While studying at the University of Göttingen, he propounded several mathematical theorems. Gauss completed his masterpieces Disquisitiones Arithmeticae and Theoria motus corporum coelestium as a private scholar. He gave the second and third complete proofs of the fundamental theorem of algebra, made contributions to number theory, and developed the theories of binary and ternary quadratic forms. Gauss was instrumental in the identification of Ceres as a dwarf planet. His work on the motion of planetoids disturbed by large planets led to the introduction of the Gaussian gravitational constant and the method of least squares, which he had discovered before Adrien-Marie Legendre published it. Gauss was in charge of the extensive geodetic survey of the Kingdom of Hanover together with an arc measurement project from 1820 to 1844; he was one of the founders of geophysics and formulated the fundamental principles of magnetism. Fruits of his practical work were the inventions of the heliotrope in 1821, a magnetometer in 1833 and – alongside Wilhelm Eduard Weber – the first electromagnetic telegraph in 1833. Gauss was the first to discover and study non-Euclidean geometry, coining the term as well. He further developed a fast Fourier transform some 160 years before John Tukey and James Cooley. Gauss refused to publish incomplete work and left several works to be edited posthumously. He believed that the act of learning, not possession of knowledge, provided the greatest enjoyment. Gauss confessed to disliking teaching, but some of his students became influential mathematicians, such as Richard Dedekind and Bernhard Riemann.

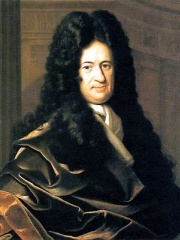
2. Gottfried Wilhelm Leibniz (1646 - 1716)

With an HPI of 84.74, Gottfried Wilhelm Leibniz is the 2nd most famous German Mathematician. His biography has been translated into 150 different languages.

Gottfried Wilhelm Leibniz or Leibnitz (1 July 1646 [O.S. 21 June] – 14 November 1716) was a German polymath active as a mathematician, philosopher, scientist and diplomat who is credited, alongside Sir Isaac Newton, with the invention of calculus in addition to many other branches of mathematics, such as binary arithmetic, and statistics. Leibniz has been called the "last universal genius" due to his knowledge and skills in different fields and because such people became much less common after his lifetime with the coming of the Industrial Revolution and the spread of specialized labor. He is a prominent figure in both the history of philosophy and the history of mathematics. He wrote works on philosophy, theology, ethics, politics, law, history, philology, games, music, and other studies. Leibniz also made major contributions to physics and technology, and anticipated notions that surfaced much later in probability theory, biology, medicine, geology, psychology, linguistics and computer science. Leibniz contributed to the field of library science by developing a cataloguing system while working at the Herzog August Library in Wolfenbüttel, Germany, that served as a model for many of Europe's largest libraries. His contributions to a wide range of subjects were scattered in various learned journals, in tens of thousands of letters and in unpublished manuscripts. He wrote in several languages, primarily in Latin, French and German. As a philosopher, he was a leading representative of 17th-century rationalism and idealism. As a mathematician, his major achievement was the development of the main ideas of differential and integral calculus, independently of Isaac Newton's contemporaneous developments. Mathematicians have consistently favored Leibniz's notation as the conventional and more exact expression of calculus. In the 20th century, Leibniz's notions of the law of continuity and transcendental law of homogeneity found a consistent mathematical formulation by means of non-standard analysis. He was also a pioneer in the field of mechanical calculators. While working on adding automatic multiplication and division to Pascal's calculator, he was the first to describe a pinwheel calculator in 1685 and invented the Leibniz wheel, later used in the arithmometer, the first mass-produced mechanical calculator. In philosophy and theology, Leibniz is most noted for his optimism, i.e. his conclusion that our world is, in a qualified sense, the best possible world that God could have created, a view sometimes lampooned by other thinkers, such as Voltaire in his satirical novella Candide. Leibniz, along with René Descartes and Baruch Spinoza, was one of the three influential early modern rationalists. His philosophy also assimilates elements of the scholastic tradition, notably the assumption that some substantive knowledge of reality can be achieved by reasoning from first principles or prior definitions. The work of Leibniz anticipated modern logic and still influences contemporary analytic philosophy, such as its adopted use of the term "possible world" to define modal notions.

3. Bernhard Riemann (1826 - 1866)

With an HPI of 79.87, Bernhard Riemann is the 3rd most famous German Mathematician. His biography has been translated into 100 different languages.

Georg Friedrich Bernhard Riemann (German: [ˈɡeːɔʁk ˈfʁiːdʁɪç ˈbɛʁnhaʁt ˈʁiːman] ; 17 September 1826 – 20 July 1866) was a German mathematician who made profound contributions to analysis, number theory, and differential geometry. In the field of real analysis, he is mostly known for the first rigorous formulation of the integral, the Riemann integral, and his work on Fourier series. His contributions to complex analysis include most notably the introduction of Riemann surfaces, breaking new ground in a natural, geometric treatment of complex analysis. His 1859 paper on the prime-counting function, containing the original statement of the Riemann hypothesis, is regarded as a foundational paper of analytic number theory. Through his pioneering contributions to differential geometry, Riemann laid the foundations of the mathematics of general relativity. He is considered by many to be one of the greatest mathematicians of all time.

4. Peter Gustav Lejeune Dirichlet (1805 - 1859)

With an HPI of 73.33, Peter Gustav Lejeune Dirichlet is the 4th most famous German Mathematician. His biography has been translated into 60 different languages.

Johann Peter Gustav Lejeune Dirichlet (; German: [ləˈʒœn diʁiˈkleː]; 13 February 1805 – 5 May 1859) was a German mathematician. In number theory, he proved special cases of Fermat's last theorem and created analytic number theory. In analysis, he advanced the theory of Fourier series and was one of the first to give the modern formal definition of a function. In mathematical physics, he studied potential theory, boundary-value problems, and heat diffusion, and hydrodynamics. Although his surname is Lejeune Dirichlet, he is commonly referred to by his mononym Dirichlet, in particular for results named after him.

5. Emmy Noether (1882 - 1935)

With an HPI of 71.70, Emmy Noether is the 5th most famous German Mathematician. Her biography has been translated into 83 different languages.

Amalie Emmy Noether (US: , UK: ; German: [ˈnøːtɐ]; 23 March 1882 – 14 April 1935) was a German mathematician who made many important contributions to abstract algebra. She proved Noether's first and second theorems, which are fundamental in mathematical physics. She was described by Pavel Alexandrov, Albert Einstein, Jean Dieudonné, Hermann Weyl and Norbert Wiener as the most important woman in the history of mathematics. As one of the leading mathematicians of her time, she developed theories of rings, fields, and algebras. In physics, Noether's theorem explains the connection between symmetry and conservation laws. Noether was born to a Jewish family in the Franconian town of Erlangen; her father was the mathematician Max Noether. She originally planned to teach French and English after passing the required examinations but instead studied mathematics at the University of Erlangen, where her father lectured. After completing her doctorate in 1907 under the supervision of Paul Gordan, she worked at the Mathematical Institute of Erlangen without pay for seven years. At the time, women were largely excluded from academic positions. In 1915, she was invited by David Hilbert and Felix Klein to join the mathematics department at the University of Göttingen, a world-renowned center of mathematical research. The philosophical faculty objected, however, and she spent four years lecturing under Hilbert's name. Her habilitation was approved in 1919, allowing her to obtain the rank of Privatdozent. Noether remained a leading member of the Göttingen mathematics department until 1933; her students were sometimes called the "Noether Boys". In 1924, Dutch mathematician B. L. van der Waerden joined her circle and soon became the leading expositor of Noether's ideas; her work was the foundation for the second volume of his influential 1931 textbook, Moderne Algebra. By the time of her plenary address at the 1932 International Congress of Mathematicians in Zürich, her algebraic acumen was recognized around the world. The following year, Germany's Nazi government dismissed Jews from university positions, and Noether moved to the United States to take up a position at Bryn Mawr College in Pennsylvania. There, she taught graduate and post-doctoral women including Marie Johanna Weiss, Ruth Stauffer, Grace Shover Quinn, and Olga Taussky-Todd. At the same time, she lectured and performed research at the Institute for Advanced Study in Princeton, New Jersey. Noether's mathematical work has been divided into three "epochs". In the first (1908–1919), she made contributions to the theories of algebraic invariants and number fields. Her work on differential invariants in the calculus of variations, Noether's theorem, has been called "one of the most important mathematical theorems ever proved in guiding the development of modern physics". In the second epoch (1920–1926), she began work that "changed the face of [abstract] algebra". In her classic 1921 paper Idealtheorie in Ringbereichen (Theory of Ideals in Ring Domains), Noether developed the theory of ideals in commutative rings into a tool with wide-ranging applications. She made elegant use of the ascending chain condition, and objects satisfying it are named Noetherian in her honor. In the third epoch (1927–1935), she published works on noncommutative algebras and hypercomplex numbers and united the representation theory of groups with the theory of modules and ideals. In addition to her own publications, Noether was generous with her ideas and is credited with several lines of research published by other mathematicians, even in fields far removed from her main work, such as algebraic topology.

6. Gottlob Frege (1848 - 1925)

With an HPI of 71.50, Gottlob Frege is the 6th most famous German Mathematician. His biography has been translated into 74 different languages.

Friedrich Ludwig Gottlob Frege (; German: [ˈɡɔtloːp ˈfreːɡə]; 8 November 1848 – 26 July 1925) was a German philosopher, logician, and mathematician. He was a mathematics professor at the University of Jena, and is understood by many to be the father of analytic philosophy, concentrating on the philosophy of language, logic, and mathematics. Though he was largely ignored during his lifetime, Giuseppe Peano (1858–1932), Bertrand Russell (1872–1970), and, to some extent, Ludwig Wittgenstein (1889–1951) introduced his work to later generations of philosophers. Frege is widely considered to be the greatest logician since Aristotle, and one of the most profound philosophers of mathematics ever. His contributions include the development of modern logic in the Begriffsschrift and work in the foundations of mathematics. His book the Foundations of Arithmetic is the seminal text of the logicist project, and is cited by Michael Dummett as where to pinpoint the linguistic turn. His philosophical papers "On Sense and Reference" and "The Thought" are also widely cited. The former argues for two different types of meaning and descriptivism. In Foundations and "The Thought", Frege argues for Platonism against psychologism or formalism, concerning numbers and propositions respectively.

7. Karl Weierstrass (1815 - 1897)

With an HPI of 70.23, Karl Weierstrass is the 7th most famous German Mathematician. His biography has been translated into 67 different languages.

Karl Theodor Wilhelm Weierstrass (; German: Weierstraß [ˈvaɪɐʃtʁaːs]; 31 October 1815 – 19 February 1897) was a German mathematician often cited as the "father of modern analysis". Despite leaving university without a degree, he studied mathematics and trained as a school teacher, eventually teaching mathematics, physics, botany and gymnastics. He later received an honorary doctorate and became professor of mathematics in Berlin. Among many other contributions, Weierstrass formalized the definition of the continuity of a function and complex analysis, proved the intermediate value theorem and the Bolzano–Weierstrass theorem, and used the latter to study the properties of continuous functions on closed bounded intervals.

8. August Ferdinand Möbius (1790 - 1868)

With an HPI of 68.90, August Ferdinand Möbius is the 8th most famous German Mathematician. His biography has been translated into 54 different languages.

August Ferdinand Möbius (UK: , US: ; German: [ˈmøːbi̯ʊs]; 17 November 1790 – 26 September 1868) was a German mathematician and theoretical astronomer.

9. Friedrich Bessel (1784 - 1846)

With an HPI of 68.69, Friedrich Bessel is the 9th most famous German Mathematician. His biography has been translated into 64 different languages.

Friedrich Wilhelm Bessel (German: [ˈbɛsl̩]; 22 July 1784 – 17 March 1846) was a German astronomer, mathematician, physicist, and geodesist. He was the first astronomer who determined reliable values for the distance from the sun to another star by the method of parallax. Certain important mathematical functions were named Bessel functions after Bessel's death, though they had originally been discovered by Daniel Bernoulli before being generalised by Bessel.

10. Ludolph van Ceulen (1540 - 1610)

With an HPI of 68.54, Ludolph van Ceulen is the 10th most famous German Mathematician. His biography has been translated into 42 different languages.

Ludolph van Ceulen (German: [ˈluːdɔlf fan ˈkɔʏlən], Dutch: [ˈlydɔl(ə) fɑŋ ˈkøːlə(n)]; 28 January 1540 – 31 December 1610) was a German-Dutch mathematician from Hildesheim. He immigrated to the Netherlands.

People

Pantheon has 106 people classified as German mathematicians born between 1013 and 1987. Of these 106, 9 (8.49%) of them are still alive today. The most famous living German mathematicians include Andrew Wiles, Robert Aumann, and Gerd Faltings. The most famous deceased German mathematicians include Carl Friedrich Gauss, Gottfried Wilhelm Leibniz, and Bernhard Riemann. As of April 2024, 12 new German mathematicians have been added to Pantheon including Johannes Werner, Johann Schreck, and Oswald Teichmüller.